Apochromatic (ED APO) lenses are designed to bring all the colors into focus in the same plane where as Achromatic lenses are corrected to bring two wavelengths into focus in the same plane.
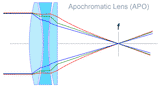
Chromatic aberration is the phenomenon of different colors focusing at different distances from a lens. Telescope objective lenses for wide-band digital imaging in astronomy must have apochromatic correction, as the optical sensitivity of typical CCD imaging arrays can extend from the ultraviolet through the visible spectrum and into the near infrared wavelength range. Apochromatic designs require optical glasses with special dispersive properties to achieve three color crossings. Explore Scientific achieves this using high-quality ED (Extra-Low Dispersion) glass from Hoya and Ohara.
| Glass Type | Code | Refractive Index nd | Abbe-number vd | Dispersion nF-nC | Refractive Index ne | Abbe-number ve | Dispersion nF'-nC' |
|---|---|---|---|---|---|---|---|
| Hoya FCD1 | 497-816 | 1.497 | 81.61 | 0.00609 | 1.49845 | 81.19 | 0.006139 |
| Hoya FCD100 | 437-951 | 1.437 | 95.1 | 0.004595 | 1.4381 | 94.66 | 0.004628 |

| Telescope | Objective Diameter mm | Focuser Size | Adapter Size | Focal Length mm | Focal Ratio | A | B | C | FULL IN Unvignetted Image Circle Diameter mm | FULL OUT Unvignetted Image Circle Diameter mm |
|---|---|---|---|---|---|---|---|---|---|---|
| 80 FCD1 metal tube | 80 | 2” | 2" | 480 | 6.0 | 46 | 51.0 | 292.0 | 26.1 | 31.0 |
| 80 FCD1 CF tube | 80 | 2” | 2" | 480 | 6.0 | 46 | 51.0 | 311.0 | 22.4 | 33.0 |
| 80FCD100 metal tube | 80 | 2.5” | 2" | 480 | 6.0 | 46 | 63.5 | 285.9 | 21.3 | 37.8 |
| 102FCD1 metal tube | 102 | 2” | 2" | 714 | 6.9 | 46 | 51.0 | 507.0 | 29.6 | 36.9 |
| 102FCD1 CF tube | 102 | 2” | 2" | 714 | 6.9 | 46 | 51.0 | 520.0 | 30.6 | 37.9 |
| 102FCD100 metal tube | 102 | 2.5” | 2" | 714 | 6.9 | 46 | 63.5 | 504.9 | 34.5 | 45.8 |
| 102FCD100 CF tube | 102 | 2.5” | 2" | 714 | 6.9 | 46 | 63.5 | 507.9 | 34.7 | 46.1 |
| 115FPL53 CF tube | 115 | 3.3” | 3" | 632 | 5.5 | 76 | 84.0 | 419.0 | 30.4 | 55.6 |
| 127FCD1 metal tube | 127 | 2” | 2" | 952 | 7.5 | 46 | 51.0 | 757.0 | 35.2 | 40.6 |
| 127FCD1 CF tube | 127 | 2” | 2" | 952 | 7.5 | 46 | 51.0 | 764.0 | 35.6 | 41.0 |
| 127FCD100 metal tube | 127 | 2.5” | 2" | 952 | 7.5 | 46 | 63.5 | 749.2 | 41.7 | 50.1 |
| 127FCD100 CF tube | 127 | 2.5” | 2" | 952 | 7.5 | 46 | 63.5 | 753.5 | 42.0 | 50.4 |
| 140FPL53 CF tube | 140 | 3.3” | 3" | 910 | 6.5 | 76 | 84.0 | 704.0 | 47.4 | 65.0 |
| 152FCD1 CF tube | 152 | 3” | 3" | 1216 | 8.0 | 76 | 76.2 | 988.6 | 51.3 | 62.0 |
| 165FPL53 CF tube | 165 | 3.3” | 3" | 1155 | 7.0 | 76 | 84.0 | 945.9 | 55.0 | 68.8 |
Note: The values listed are for the image circles with the focuser drawtube inserted all the way (worst case) and pulled all the way out (best case)
User Focus Position Circle Calculation
Using value A, B, C, and the Focal Length from the table, calculate your specific focus position image circle using the position as read off your drawtube:
A = Focus Travel Length (mm)
B = Drawtube Inside Diameter (mm)
C = Length between main objective and end of drawtube (fully inserted)
Procedure
1. Using your image configuration, focus your camera.
2. Read the focus position (mm) on the drawtube.
3. Calculate your image circle = B* ((C-A) + step 2 value)/Focal Length
Example:
For the 80 FCD1 with a reading of 35 mm from the drawtube:
Image Circle = 51.0 * (((292.0 - 46) + 35)/480)
= 51.0 * (281/480)
= 29.9 mm

